What Is A Learning Curve?

A learning curve is a mathematical representation of the relationship between cost and output that takes place in a repetitive task over a period of time.

It was first described by Hermann Ebbinghaus in 1885, and it is used to measure performance efficiency over time and to predict costs.

It is usually plotted with the vertical axis representing learning, productivity, or efficiency, and the horizontal axis showing the time or number of trials spent on the activity. Figure 1 shows a typical s-shaped learning curve.

Figure 1: s-shaped learning curve

Learning curves are widely used in machine learning and deep learning, where performance is represented by the accuracy or error of the learning system, and experience by the number of iterations used to obtain an optimal solution. Usually, data scientists plot the loss function calculated on the validation dataset versus the loss function calculated on the training dataset in order to determine model performance.

Why Is The Learning Curve Important?

In machine learning, the learning curve is useful for many purposes, like comparing the performance of different models, adjusting parameters to obtain model convergence, determining the optimal size of the training dataset, and detecting overfitting and underfitting. For example, figure 2 shows the overfitting of a model, explained by the fact that the validation and training loss functions increasingly diverge as the amount of trials increases.

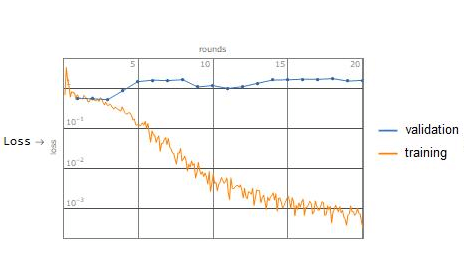
Figure 2: learning curves showing overfitting.

Source: Dennis, CC BY 4.0, via Wikimedia Commons

Learning Curve and LogicPlum

LogicPlum’s platform is a modeling tool based on machine learning, statistics, and artificial intelligence. It estimates learning curves in many of its processes to optimize dataset size, compare algorithms, provide better convergence, and more.

However, to reach an optimal model requires training and comparing many possible solutions. This is where LogicPlum’s platform competitive advantage resides: through automation, it trains and evaluates hundreds of algorithms, and then selects the optimal one according to a specified metric. In this manner, users don’t need expertise in mathematics and statistics, but only the capacity to understand and interpret the model’s results.

Additional Resources

• Wikipedia. (2020). Learning curve (machine learning). Available at https://en.wikipedia.org/wiki/Learning_curve_(machine_learning)