What is a Continuous Probability Distribution?
A continuous probability distribution is the distribution of a continuous random variable. As the random variable is continuous, it can assume any number from a set of infinite values, and the probability of it taking any specific value is zero. Therefore, statisticians use ranges to calculate these probabilities.
The probability that a random variable X falls within the range [a,b], with a continuous probability function f(X) is given by the probability density function, which is defined as:
![]()
An example of a continuous probability distribution is the normal distribution, which is defined as:
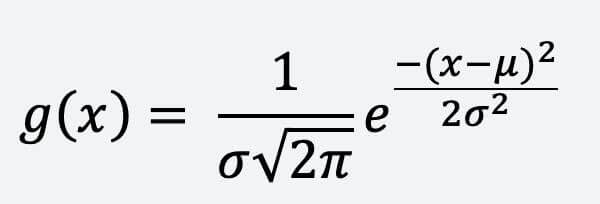
where μ is the mean and σ the variance.
Moreover, there are many well-known continuous probability distributions. Some of the most common ones are the Beta distribution, which is used to estimate success probabilities; the Kumaraswamy distribution, which is similar to the Beta distribution but easier to handle; the Marchenko–Pastur distribution, which is applied in the theory of random matrices; and many more.

Figure 1: Kumaraswamy Probability density function
Source: Krishnavedala, CC0, via Wikimedia Commons
Why is the Continuous Probability Distribution Important?
Continuous probability functions find a large number of applications, the most commonly used being the normal distribution. This type of distribution is widely used in statistical quality control, research studies, engineering calculations, geological surveys, political analysis, medical tests, and many more.
Continuous Probability Distribution + LogicPlum
Although the concept of a continuous probability distribution can be easily understood, its application may require difficult calculations. Besides, deciding what continuous distribution function to use requires expert knowledge, which is not always in the realm of every analyst.
LogicPlum’s platform is a tool that helps everyone to create statistical and machine learning models, without requiring the necessary mathematical knowledge. It provides this service by doing all the modeling steps in an automated manner and providing its users with a report explaining all the operations done.
In this manner, users can concentrate on interpreting results and producing forecasts in their fields of expertise, being assured that they are employing the latest mathematical and statistical tools.
Guide to Further Reading
Wikipedia. Probability Distribution. Continuous Probability Distribution. Available at https://en.wikipedia.org/wiki/Probability_distribution#Continuous_probability_distribution








