Discriminant
What is a Discriminant?
In mathematics, the discriminant is a parameter of a system that is calculated to help its classification or solution. It is widely used in many areas of this science, such as factoring polynomials, number theory, and algebraic geometry. Its name was coined by British mathematician James Joseph Sylvester in 1851.
Mathematically, the polynomial discriminant can be defined as:
Given a polynomial A(x) = a0 + a1 x + a2 x2 + … + an xn and its derivative A’(x) = a1 + a2 x + … + ann xn-1, we define the discriminant of A as
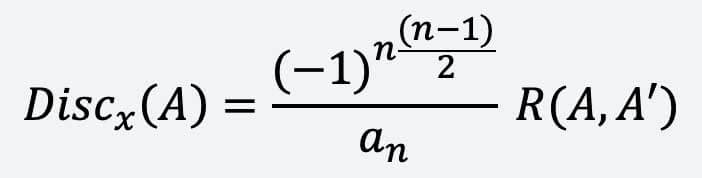
where R stands for resultant.
Thus, for second degree polynomials the discriminant is D2 = a12 – 4a0a2 and for a third degree polynomial the discriminant is D3 = a12a22– 4a0a23 – 4a13a3 + 18a0a1a2a3 – 27a02a32.
Generally speaking, the discriminant of a polynomial of positive degree equals zero if and only if the polynomial has a multiple root. If the discriminant is different from zero, the polynomial has real and complex roots.
The discriminant concept has been generalized to other areas of mathematics. For example, to the discriminant of an algebraic number field and the discriminant of a form.
Why is the Discriminant Important?
The discriminant is a mathematical concept that is used in many fields, such as binary quadratic forms, elliptic curves, metrics, quadratic fields, and the second derivative test. As such, it has an important role not only in the algebra of polynomials but also in mathematical analysis, neural networks, and deep learning techniques.
Discriminant + LogicPlum
Modeling requires many mathematical and statistical concepts and methods. One of them is the discriminant, which is usually used as part of different calculations. Although a simple concept, its calculation can become complex.
This problem is solved by LogicPlum’s platform through automation, where all algorithms are resolved without the need for human intervention. As a result, hundreds of possible solutions to a model can be tried in a short time and the optimal one selected according to a specified criterion.
Additional Resources
A good source for mathematical definitions is
Wolfram MathWorld. Available at https://mathworld.wolfram.com/








