Logistic Function

What is the Logistic Function?

The logistic function is a mathematical function that is mainly used to model the growth of a population. Mathematically it is expressed as

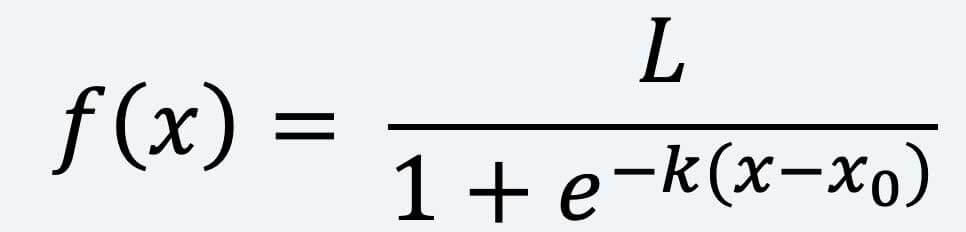
Its main characteristic is its S shape, which represents a slow growth at the beginning that then speeds up until it reaches a limit value of A. Figure 1 shows the logistic function when L= k = 1 and x₀ = 0.

A generalization of the logistic function is the hyperbolastic function of type I.

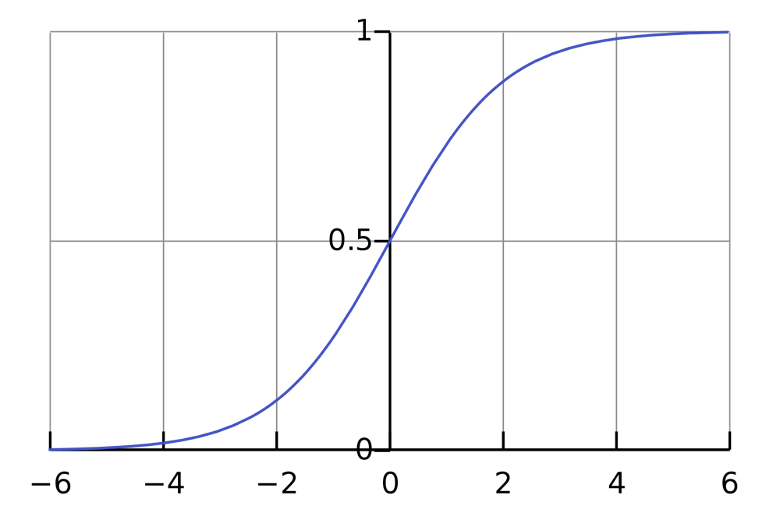
Figure 1 – Logistic Function
Source: Qef, Public domain, via Wikimedia Commons

The logistic function was introduced by Pierre-François Verhulst in 1838 in a series of three papers published between 1983 and 1847. The equation was developed to explain situations of limited growth, which counteracted Malthus’ idea of unconstrained growth.

Why is the Logistic Function Important?

The logistic function has a wide range of applications that encompass many fields, such as biomathematics, chemistry, physics, demography, economics, finance, geoscience, mathematical psychology, oil industry, probability, sociology, political science, linguistics, statistics, and artificial neural networks.

For example, in physics, the logistic function models the statistical distribution of fermions over the energy states of a system in thermal equilibrium. Similarly, in linguistics, it is used to explain language change; and in statistics, this function is the base of the logistic regression model, which is used in classification to define a pass/fail test.

The logistic function is also used in neural networks to model nonlinearity and to limit signals to within a specified interval.

Logistic Function + LogicPlum

The logistic function is used in many of LogicPlum’s machine learning algorithms. It provides a way to model a non-linear, constrained growth that applies to many problems. LogicPlum’s platform provides the possibility of testing many different variations of the logistic function in a very short time and without the need for deep mathematical knowledge. As a result, an efficient solution can be found in a cost-effective way.

Additional Resources

Cinema M119. (2013).  Section 4.7 – Introduction to Logistic Functions   Available at https://www.youtube.com/watch?v=hois2F0bSxU